The Golden Mean

The question of what natural, pleasing harmony was in terms of proportions, and whether pictorial harmony could be expressed in phrased definitions or in mathematical formulas or in geometrical constructions, was a puzzle that occupied mathematicians and natural philosophers since old. The laws of harmony could indeed, it was thought, hide some of the knowledge of the divine.

The Golden Mean in Algebra

Two mathematicians thought they could provide answers. In the beginning of the thirteenth century, the mathematician Leonardo of Pisa (1175-1240), called Fibonacci, defined a series of numbers, in which the first two numbers were one, and in which each subsequent number is the sum of the two preceding numbers. This series is now rightly called the Fibonacci series. The numbers are:

1 ... 1 ... 2 ... 3 ... 5 ... 8 ... 13 ... 21 ... 34 ... 55 and so on.

The mathematical formula to calculate the numbers of such a series is

A₁=1, A₂=1 and from n=3 counts that A_n = A_n-1 + A_n-2

Another formula that generates this series is the formula of Edouard Lucas

An = (1/{sqrt5})( [(1+{sqrt5})/2]ⁿ - [(1-{sqrt5})/2]ⁿ )

In this formula {sqrt5} stands for the square root of 5.

The ratio X = (1+{sqrt5})/2 is called the Golden Number. Its value is 0.618.

The reciprocal Y = (1-{sqrt5}/2]ⁿ is also considered as an important number, and its value is 1.618.

One obtains the Fibonacci series by adding numbers. One can also divide the numbers of this series to obtain proportions. The proportions of the numbers from the fourth number on are proportions that are therefore presumed also to be special proportions: 5/3 or 8/5 and so on. These proportions almost, but not exactly follow the formula of the Golden Number. The proportion of two subsequent numbers of the Fibonacci series (such as 3/3, 5/3, 8/5 and so on) tends to 1.618. It is not exactly 1.618, as figures continue indefinitely after the last figure mentioned here, after the figure 8.

In the Fibonacci series above, we used as starting numbers two times the number one, but any series starting by any two arbitrarily chosen numbers and built by the rule A_n = A_n-1 + A_n-2 has the property that the proportion of two adjacent generated numbers tends to the value 1.618. This number also is often called the Golden Mean.

The Fibonacci series has other remarkable properties. For instance, the series starting with the numbers 1 and 1.618… is special. The series is:

1 ... 1.618… ... 2.618…. ... 4.236… ... and so on.

In this series not only A_n = A_n-1 + A_n-2 but also A_n = A_n-1 * 1.618… so that if we name x=1.618…. the series is also:

1 ... x ... x² ... x³ ... and so on.

So this series is at the same time a mathematical series (each number is obtained by adding preceding numbers) and a geometrical series (each number is obtained by a multiplication of preceding numbers).
The Fibonacci series based on 1 and 1.618… is the only series that exists that has these strange, remarkable properties. Therefore, people spoke not only of Golden Mean and Golden Proportions, but also of Divine Proportion.

The Golden Mean in Geometry

There is an equivalent of the Divine Proportion in geometry. In the fifteenth century another Italian mathematician, Luca Pacioli, defined a Divine Proportion as a segment of a line divided into such parts that the smaller part’s length A is to the larger part B as the larger part is to the entire segment’s length (A + B). This is also called the Golden Section.

In formula that means that A/B=B/(A+B) with A the shortest part.

The connection with the Fibonacci series is in that when one substitutes (A+B) = 1 in the previous formula one obtains a second-degree equation

B² + B – 1 = 0

And the double solution of this equation is

B₁ = - (1 - {sqrt5})/2 and B₂ = - (1+ {sqrt5})/2

In which one finds back the Golden Number and its reciprocal.

Therefore, the numbers of the Fibonacci series and the Golden Mean seem to be connected.

An infinite number of lengths comply with the rule that A/B=B/(A+B), but as integers we find that only the adjacent numbers of a Fibonacci series are values that approximately satisfy the rule.

The true Golden Section can be easily constructed for any piece of line of length (A+B).
Let us call this line as determined by two endpoints a and b. In b draw a line perpendicular to ab. On this perpendicular take the length C = ab/2. In the endpoint c draw a circle of radius ab/2. Now draw the line ac. Where this line ac cuts the circle, one has a point d. The length ad is the length that divides the line ab in the Golden Section. See the next plate (plate 64). The proportion ab/ad equals 1.618…, the Golden Mean!

The Fibonacci proportions were found to best represent harmonious proportions, proportions that humans find pleasing and attractive.

The proportions of the Golden Mean were quite popular from the Middle Ages on to our times and they remain considered as the main and basic proportions of harmony. Rectangles with these proportions are presumed to be the most aesthetically pleasing. Thus they were called divine proportions. These proportions can be found back in many pictures, whether knowingly designed or intuitively introduced by painters.

The proportions can be used in the design of the areas of composition. See the next plate. By always dividing an area according to the proportions of the golden mean, one obtains a progression of ever-smaller surfaces. Such areas and constructions are found in nature and can also be the basis for design of basic areas of composition in pictures, for areas in which separate scenes can be positioned. It is rare however to find more than one or two of such areas in paintings since the areas rapidly grow too small. The areas can be easily constructed by starting with one vertical golden mean division, drawing the diagonal and then a horizontal line where the diagonal meets the vertical, and so on. See plate 65.

The Golden Mean in Art

Painters have often used the Divine or Golden Proportions in their work. The following plate (plate 66) shows a frame with (almost) Golden Mean proportions. This is then called a Golden Rectangle.

Painters have used these proportions because they are pleasing to humans. The most classic example of a picture constructed upon complex geometrical patterns is Piero della Francesca’s "Baptism of Christ". In the figure hereunder we show some of the lines of the painting. The Golden Section proportions and the Fibonacci numbers can be found about anywhere in this picture

Piero took a frame that he divided in three horizontal parts. The number three stands as the first number in the Fibonacci series, and also had importance for Piero as being the number of the Trinity in his Roman Catholic religion, that is the three aspects of God as Father, Son and Spirit. The top part he turned into half a circle. The median line of that circle passes through the symbol of the Holy Spirit, the dove. The other horizontal line that divides the lower, rectangular part of the panel in two halves passes through Jesus’ navel. The rectangular part of the panel thus covers 2/3 and that is the first proportion of the Fibonacci numbers too.

The outside, global measures of the panel are a Fibonacci proportion, so that the panel is a golden rectangular. The panel is divided in two vertical parts. The vertical median line passes exactly in the middle of Jesus, through the middle of his folded hands, and also through the middle of the dove representing the Holy Spirit.
The tree on the left stands around a middle line and this line is at a 3/5 distance of the middle vertical of the panel.
On the right too, a vertical can be thought to pass almost through the middle of John the Baptist. This line is more the middle of the Baptist’s right leg, and this line then again lies at a 3/5 distance from the right border of the panel.

Lengths and heights also follow Fibonacci number proportions. The height of the white angel that stands in the middle of the three in the left group is about 3/5 of the height of the rectangular part of the panel (which itself is 2/3 of the total height). There are three angels here, not four and not two; remember the Trinity. Jesus’ body bears the same proportions, but not when his head is counted into the number.

Jesus holds his arms together in prayer. One can draw a triangle from the middle of the Holy Spirit symbol along Jesus’s elbows down to the lower border. In that triangle again one finds Fibonacci proportions.
Two further triangles can be drawn. One starts at the feet of Jesus, and goes upwards to the two corners of the rectangular of the panel. The same triangle, but the inverse triangle, starts from the middle of the cup above Jesus’s head and goes down to the two lower corners of the panels. These two triangles intersect at a horizontal passing through Jesus’ navel again.

All these lines are too many to have accumulated by chance. Piero must indeed have drawn lines first, and build his composition upon them. He applied the Golden Mean in pany places. Piero della Francesca was a mathematician who abandoned painting later on to write a treatise on perspectives. He based his lines on numbers, in the spirit of the late Middle Ages as it was obsessed with a search for absolute, perhaps holy numbers.

Look at Piero della Francesca’s structure of the "Baptism of Christ" in the following plate (plate 67):

Piero della Francesca may have used the proportions 1/3, 2/3 and 3/5 also simply because they make sense aesthetically.
For instance, when one paints a group of people and lets an open space next to them, then dividing the space horizontally in 2/3 gives two proportions for the group and one next to the group. This is a bit too heavy, so a 3/5 is almost right, and a painter may well come to this 60% instead of 66% or 75% in a quite natural "eye’s" way.
It is always difficult to tell whether indeed exact numbers have been used or not by painters or whether they arrived at a certain distribution of forms by intuition, and then came to proportions that are close to the Fibonacci numbers. Even in Piero’s painting we can be sure of certain lines and proportions, but others may have come to us purely by the artistic feeling of the painter.

-> Piero della Francesca (1410/1420 – 1492). The Baptism of Christ. The National Gallery – London. Ca. 1460.

Another of Piero’s paintings, the "Flagellation of Christ", is also an obvious example of scenes ordered according to Golden Mean divisions.
The picture consists of two scenes. The scene on the right may represent Italian noblemen and merchants arguing on their business and thereby turning their backs, ignoring the scene on the left side. Here, soldiers flagellate Christ at a column. Seated in front of Christ is not Pilate however, but most probably the last Byzantine Emperor Constantine Paleologus, who is forced to assist to a scene of torture of Christ staged by the Turkish sultan Mohammed II, who conquered Christian Constantinople in 1453.
Piero della Francesca had seen the previous Byzantine Emperor participating in the Papal Council of Florence. That Emperor had asked help from the Italian city-states against the Ottoman pressing army. The Council discussed the main issue between the Catholic and Orthodox churches, the "Filioque" term, which defined in the Nicene Creed the nature of the Trinity.
The lengths of the two scenes of the painting are in the proportion of the Golden Mean. The left scene then contains again two parts, horizontally and vertically. In the left scene stands the column against which Christ is being flagellated, and this column divides the left scene in two sub-scenes, the lengths of which are in the Golden Mean. The left scene is horizontally divided in two parts. One part contains the scene with the figures, the other the ceiling.
Furthermore, the right scene of the painting holds three figures, and the right scene holds five figures, which are Fibonacci numbers.

-> Piero della Francesca (1410/1420 – 1492). The Flagellation of Christ. Galleria Nazionale delle Marche. Urbino. After 1459.

The next plate 68 shows the Structure of Piero della Francesca’s "Flagellation of Christ".

The Golden Mean continued to fascinate also modern artists. An artist of the Italian "Arte Povera" movement of the twentieth century, Mario Merz, was so fascinated by the quasi mystic qualities of the Fibonacci numbers, that he made them to be the major subject of a series of sculptures and paintings.
In his work "Dusk in a little Cup", Merz joined to his canvas electric gas tubes that emit a blue light to represent the Fibonacci numbers, and more numbers are painted on the image of the cup.

-> Mario Merz (1925 - ). Dusk in the little Cup. Museo Nacional Centro de Arte Reina Sofia. Madrid. 1979.

The Golden section remained in the memories of painters, and appeared in unexpected subjects.
Johannes Itten (1888-1967) wrote about the spatial effect of colours. He noted that some colours by their hue quality seem to be closer to viewers than others. Yellow for instance was a colour that seemed close to viewers, blue kept a distant to the viewer.
Itten stated that the six fundamental hues on a black ground conformed to the Golden Section in their gradation of depth ^G95. He argued that when the colour orange is interposed between the interval of depth between yellow and red, then the intervals between yellow and orange related to the interval between orange to red as the minor to the major part of the Golden Section. The same proportions were valid for yellow to red-orange and from red-orange to blue and also from yellow to red and red to violet, from yellow to green and green to blue, and so on.

Painters used the Golden Mean to split their scenes in sub-scenes, as we saw with Piero della Francesca. The Golden Number has also been used in positioning a particular attention item in a painting. Where would a painter place a human figure, a tree, a prominent building in a landscape? Placing such an important element of vision straight in the middle would be too dull. Somewhat to the left, or somewhat to the right of the central point, seemed indicated. But how much from the centre should a painter position a prominent feature? The Golden Section provided a good answer, and moreover one that had received a connotation of mystery. The Golden section distances have thus often been used by painters to create a pleasing and relaxed impression on important elements of their pictures.

The point that lies at the crossing of the Golden Section applied on the two borders of a rectangular frame is called the Golden Point of the frame. In fact, there are four such points, depending on the combinations of how the golden section is applied on each border line. Often a viewer will find in one of these points a prominent pictorial element of the painting, such as a human figure, a plant, a building, a vanishing point of perspective.

The proportions such as 3/2, 5/3, and 8/5 are easy to use by painters in their frame. These approximate the Golden Mean. By using these easier sections in a frame one obtains a cloud of points around the true Golden Points. So, painters could as well just stand before a rectangular canvas, point with their brush to a place some distance away from the centre, and start to design their main viewing element at such a point. In this way, they used their intuition to create something pleasing. Later only, it was found that the Golden Section and the Golden Mean corresponded to such positions. And when painters learnt about the Divine Proportion, of course, they started to use the proportion for good and on purpose.
So, the Golden Section is merely a geometrical construction, and the Divine Proportion a mathematical number that coincides with a pleasing effect. Or is there more, and really something mysterious to the number?

The Golden Mean in Nature

The psychologist Rudolf Arnheim noted, "Traditionally and psychologically, this proportion of 1:0.618 has been considered particularly satisfying because of its combination of unity and dynamic variety. Whole and parts are nicely adjusted in strength so that the whole prevails without being threatened by a split, but at the same time the parts retain some self-sufficiency ^G96 ."

If we would only find the divine proportion in human-devised geometric structures of pictorial composition or in architectures, the concept of the Golden Mean would ever stay artificial, and merely be a product of our mathematical minds. The proportions of the Golden Mean have however also been found in nature, wherever people searched for harmonious proportions. The proportion was found in the progressions of the measures of the spiral houses of sea molluscs, in patterns of flowers, in proportions of lengths of limbs of animals.

And of course, the Golden Number was found in proportions of features of the human body!
Painters and philosophers wondered since long when a body would be attractive or less so, or when a human face could be called attractive. It seems that the Golden Mean provides part of the answer.
The human body is attractive when it is symmetric, and when the proportions of its lengths and widths obey in its several measures the Golden Mean. A fine body has legs that are 1.618 times longer than its rump. The figure of a woman whose proportion of bust and waist approaches 1.618 is considered fine.
Furthermore, a face is fine when Golden Mean proportions rule its traits. Girls have fine faces for instance, when the proportion of the length of their mouth to the width of their nose is about 1.618. Various other Golden Mean proportions can be defined on the human face. And also in other features of attractive faces these proportions can be measured regularly. No wonder that Renaissance artists devised the ideal man based on Golden Mean proportions.
Whether this is fact or all fiction is a difficult question, but the coincidence is often and repeatedly striking. A fine face of course has to be the face of a healthy youth, and probably also has to remind us of very young children that are so appealing to our help. But it really seems that attractiveness could be explained in terms of symmetry, balance and 1.618.

The Golden Mean is a mysterious proportion. It was defined and described by a Middle Age mathematician, but it appeals to humans in strange, and as yet unexplained ways. And it may hold, partially at least, the clue of why humans find some of their own faces and bodies beautiful.

Back to the navigation screen (if that screen has been closed)

Last updated: May 2010

Book

Copyright: René Dewil - All rights reserved. The electronic form of this document is copyright. Permission is granted for electronic copying, distribution in print form for educational purposes and personal use. If you do reduplicate the document, indicate the source as 'René Dewil - The Art of Painting - Copyright'. No permission is granted for commercial use and if you would like to reproduce this work for commercial purposes in all or in part, in any form, as in selling it as a book or published compilation, then you must ask for my permission formally and separately.
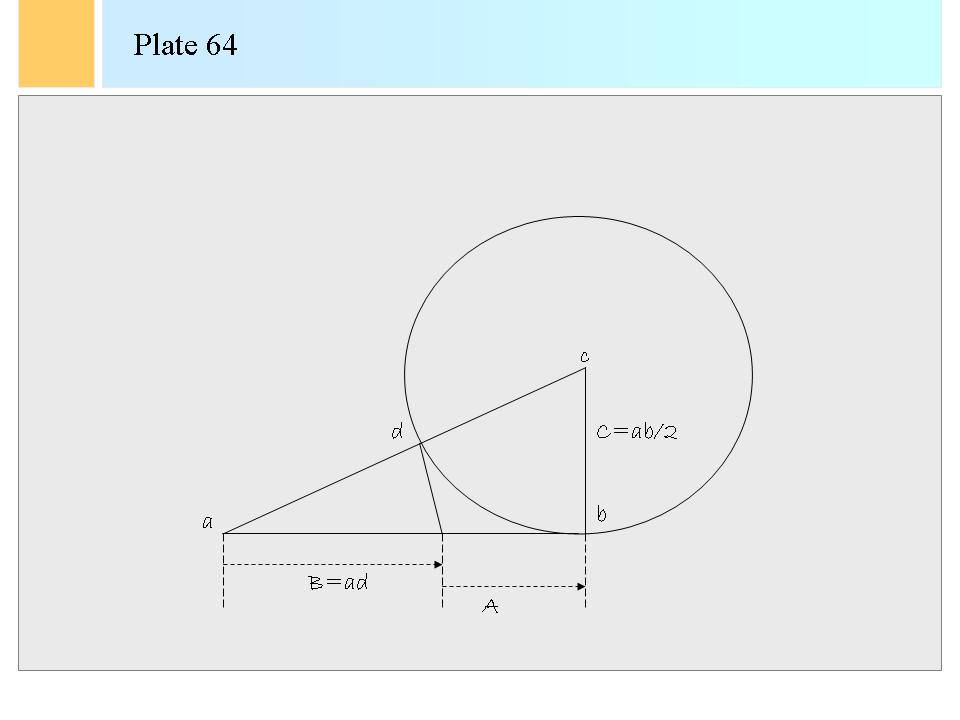
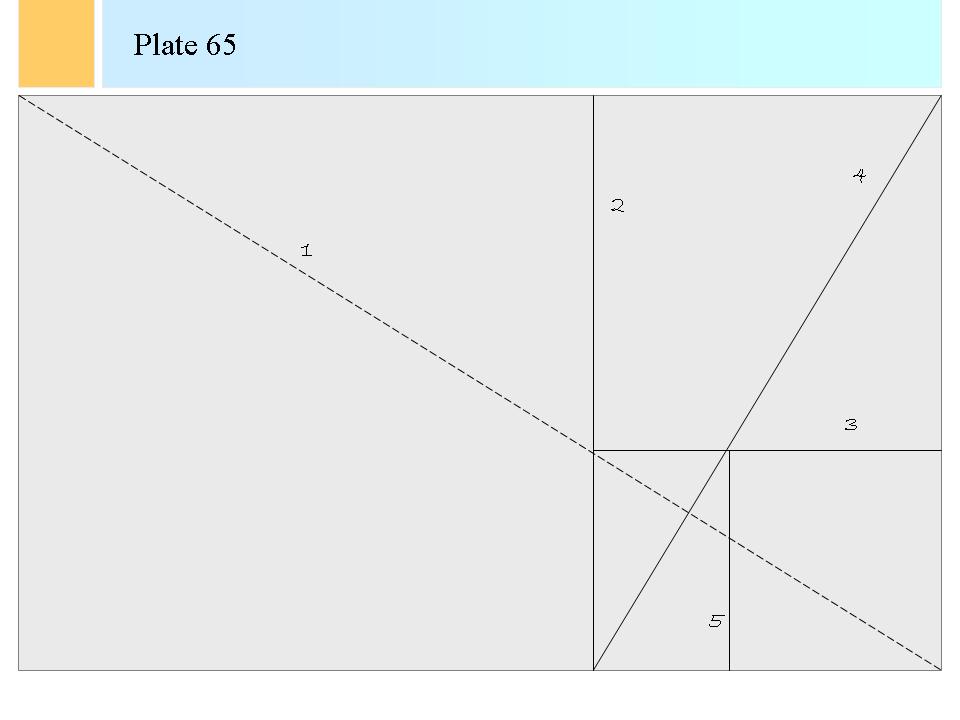
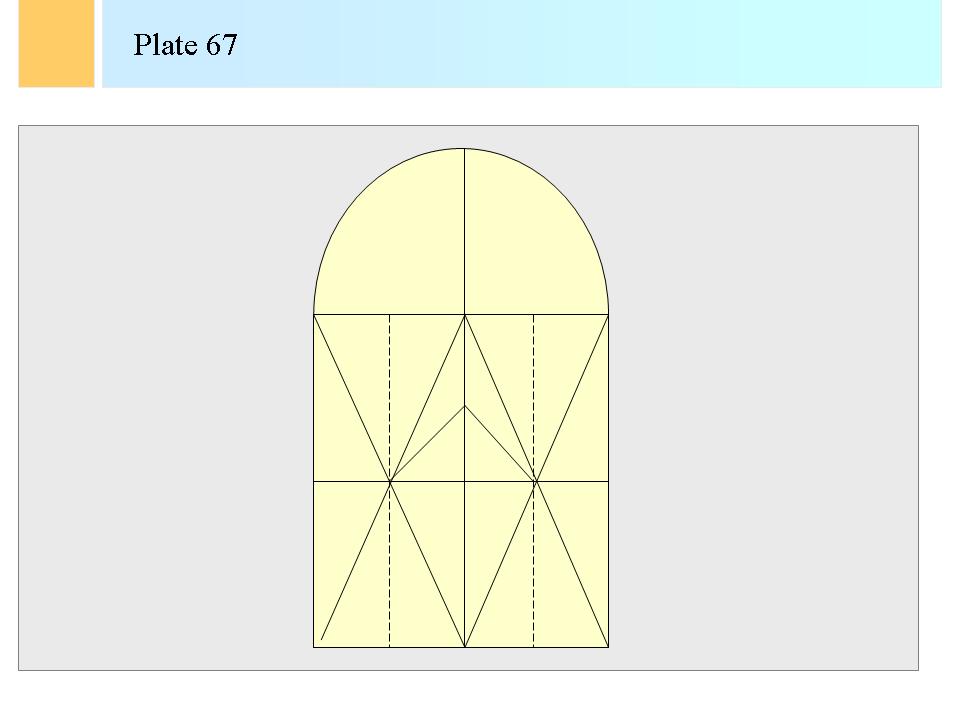